Skip to main content

Introduction to Calculus

speedometer
Sam and Alex are travelling in the car ... but the speedometer is broken.
Alex:
"Hey Sam! How fast are we going now?"
Sam:
"Wait a minute ..."
"Well in the last minute we went 1.2 km, so we are going:"
1.2 km per minute x 60 minutes in an hour = 72 km/h
Alex:
"No, Sam! Not our average for the last minute, or even the last second, I want to know our speed RIGHT NOW."
Sam:
"OK, let us measure it up here ... at this road sign... NOW!"
road
"OK, we were AT the sign for zero seconds, and the distance was ... zero metres!"
The speed is 0m / 0s = 0/0 = I Don't Know!
"I can't calculate it Sam! I need to know some distance over some time, and you are saying the time should be zero? Can't be done."

That is pretty amazing ... you'd think it is easy to work out the speed of a car at any point in time, but it isn't.
Even the speedometer of a car just shows us an average of how fast we were going for the last (very short) amount of time.

How About Getting Real Close

But our story is not finished yet!
Sam and Alex get out of the car, because they have arrived on location. Sam is about to do a stunt:
jump t=1

Sam will do a jump off a 20 m building.

Alex, as photographer, asks:

"How fast will you be falling after 1 second?"

Sam uses this simplified formula to find the distance fallen:
d = 5t2
  • d = distance fallen, in metres
  • t = time from jump, in seconds
(Note: the formula is a simpler version of how fast things fall under gravity: d = ½gt2)
Example: at 1 second Sam has fallen
d = 5t2 = 5 × 12 = 5 m
But how fast is that? Speed is distance over time:
Speed =distance
time
So at 1 second:
Speed =5 m= 5 m/s
1 second
"BUT", says Alex, "again that is an average speed, since you started the jump, ... I want to know the speed at exactly 1 second, so I can set up the camera properly."

jump from t=1 to t=1
Well ... at exactly 1 second the speed is:
Speed =5 − 5 m=0 m= ????
1 − 1 s0 s
So again Sam has a problem.
Think about it ... how do we figure out a speed at an exact instant in time?
What is the distance? What is the time difference?
They are both zero, giving us nothing to calculate with!
But Sam has an idea ... invent a time so short it won't matter.
Sam won't even give it a value, and will just call it "Δt" (called "delta t").
So Sam works out the difference in distance between t and t+Δt
At 1 second Sam has fallen
d = 5t2 = 5 × (1)2 = 5 m

At (1+Δt) seconds Sam has fallen
d = 5t2 = 5 × (1+Δt)2 m

We can expand (1+Δt)2:
(1+Δt)2= (1+Δt)(1+Δt)
= 1 + 2Δt + (Δt)2

And we get:
d= 5 × (1+2Δt+(Δt)2) m
= 5 + 10Δt + 5(Δt)2 m

jump from t=1 to t=1 + delta t

So between 1 second and (1+Δt) seconds the distance fallen is:
Change in d= (5 + 10Δt + 5(Δt)2) − 5 m
= 10Δt + 5(Δt)2 m

Now divide that distance by time to get the speed:
Speed= 10Δt +5(Δt)2 mΔt s
= 10 + 5Δt m/s

So the speed is 10 + 5Δt m/s, and Sam thinks about that Δt value ... he wants Δt to be so small it won't matter ... so he imagines it shrinking towards zero and he gets:
Speed = 10 m/s

Wow! Sam got an answer!

Sam: "I will be falling at exactly 10 m/s"
Alex: "I thought you said you couldn't calculate it?"
Sam: "That was before I used Calculus!"

Yes, indeed, that was Calculus.
small stones
The word Calculus comes from Latin meaning "small stone".
Because it is like understanding something by looking at small pieces.
· Differential Calculus cuts something into small pieces to find how it changes. 
· Integral Calculus joins (integrates) the small pieces together to find how much there is.
(And Differential Calculus and Integral Calculus are like inverses of each other, just like multiplication and division are inverses.)

Sam used Differential Calculus to cut time and distance into such small pieces that a pure answer came out.

So ... was Sam's result just luck? Does it work for other things?
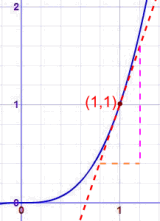
Let's try doing this for the function y = x3
This is going to be very similar to the previous example, but it will be just a slope on a graph, no one has to jump for this one!

Example: What is the slope of the function y = x3 at x=1 ?


At x = 1:y = 13 = 1
At x = (1+Δx):y = (1+Δx)3

We can expand (1+Δx)3 to 1 + 3Δx + 3(Δx)2 + (Δx)3, and we get:
y = 1 + 3Δx + 3(Δx)2 + (Δx)3

And the difference between the y values from x = 1 to x = 1+Δx is:
Change in y= 1 + 3Δx + 3(Δx)2 + (Δx)3 − 1
= 3Δx + 3(Δx)2 + (Δx)3

Now we can calculate slope:
Slope =3Δx + 3(Δx)2 + (Δx)3Δx
= 3 + 3Δx + (Δx)2

Once again, as Δx shrinks towards zero we are left with:
Slope = 3
And here we see the graph of y = x3

The slope is continually changing, but at the
point (1,1) we can draw a line tangent to the curve
and find the slope there really is 3.
(Count the squares if you want!)
graph x^3 slope at (1,1)
Question for you: what is the slope at the point (2,8)?

Try It Yourself!

Go to the Slope of a Function page, put in the formula "x^3", then try to find the slope at the point (1,1).
Zoom in closer and closer and see what value the slope is heading towards.

Conclusion

Calculus is about changes.
Differential calculus cuts something into small pieces to find how it changes.
Integral calculus joins (integrates) the small pieces together to find how much there is.

Comments

Post a Comment

Popular posts from this blog

How do I use lucky patcher without rooting the phone?

Image
Someone requested me to describe the whole process.Here is how we do it. 1. First download  Lucky Patcher. 2.Install it in your phone. 3.Open the app.you will see something like this. 4.Click on the app you want to modify.Like i selected hill climb racing 5.Click on "Open menu of patches" 6.Now click on "create modified apk" 7.If custom patch is available click on it.If not click on"Apk rebuilded for InApp and LVL emulation".Here I am clicking on "Apk rebuilded for InApp and LVL emulation". 8.Click on "Rebuild The App" & wait some time 9.If it shows patch pattern success.Then there are chances if it shows all failed means there are no chances of you cracking the app.Here we got patch pattern success. 10.Click on Go to file. 11.Click on the file.Select Uninstall & install 12.It  will ask you to uninstall the installed app.Go on uninstall the app.After unistalling cancel it.
Read more

A list of phobias.....

Image
We define a phobia as ‘an extreme or irrational fear of or aversion to something’. You are probably aware of the more common phobias, such as  arachnophobia  (fear of spiders),  claustrophobia  (fear of enclosed spaces), and  agoraphobia  (fear of open places), but did you know there are also words which describe the fear of  idleness ,  worms , and even  body odour ? Explore the list of phobias below to discover the terms for some of the more interesting fears you may encounter. Although if you suffer from  logophobia  (a fear of words), it’s probably best to step away from this blog now… in fact, this list of phobias may be your worst nightmare! OBJECT PHOBIA air travel aerophobia American people and things Americophobia animals zoophobia bacteria bacteriophobia beards pogonophobia beating mastigophobia bed clinophobia bees apiphobia birds ornithophobia blood haemophobia blushing erythrophobia body odour bromidrosiphobia bridges gephyrophobia bullets ballistoph
Read more

Erythrocytes

Image
The  erythrocyte , commonly known as a red blood cell (or RBC), is by far the most common formed element: A single drop of blood contains millions of erythrocytes and just thousands of leukocytes. Specifically, males have about 5.4 million erythrocytes per microliter ( µ L) of blood, and females have approximately 4.8 million per  µ L. In fact, erythrocytes are estimated to make up about 25 percent of the total cells in the body. As you can imagine, they are quite small cells, with a mean diameter of only about 7–8 micrometers ( µ m). The primary functions of erythrocytes are to pick up inhaled oxygen from the lungs and transport it to the body’s tissues, and to pick up some (about 24 percent) carbon dioxide waste at the tissues and transport it to the lungs for exhalation. Erythrocytes remain within the vascular network. Although leukocytes typically leave the blood vessels to perform their defensive functions, movement of erythrocytes from the blood vessels is abnormal. Shape an
Read more